The antilog tables

`69.31\times 57.43`

Is the same as `10^1.8408\times 10^1.7591` (see previous pages)

Add the indices:

First make the sum before looking at the antilog table

This equals `10^3.5999`

So how do we convert `10^3.5999` back to a normal number?

We can either use

1. Antilog tables

or

2. log tables in reverse

Antilog tables

Antilog `10^3.5999`

Antilogs only work between 0 and 1

`10^3.5999` = `10^3\times10^0.5999`

`10^3 = 1000`

And we must look up `0.5999`

Here is part of the antilog tables:

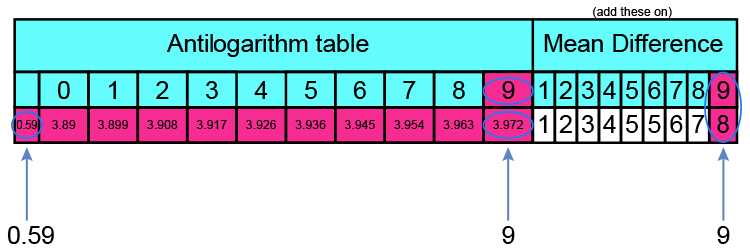
First look up 0.59999 on the anti log table

`3.972 + 8 ` (to the last digit) `= 3.980`

Antilog table of `0.5999 = 3.980`

So `10^3.5999 = 10^3\times 10^0.5999`

Or `10^3.5999 = 1000\times 3.980`

` = 3980`

NOTE:

There is one other way to look up the antilog figure if you don’t have an antilog table and that is to use the log tables in reverse.

Antilog tables = Log tables in reverse

Here is part of the log tables:

Anti logarithm tables are logarithm tables in reverse

`0.5999 = 3.980`

(So this provides the same answer)