The log tables

So, how did we convert `69.31\times57.43` into `10^(1.8408)\times10^(1.7591)` ?

We used log tables (see reference log tables above)

Look up `69.31`

Logs only work between `1` and `10`

And so `69.31` is `10^1\times6.931`

So we look up `6.931`

Here is part of the log table:

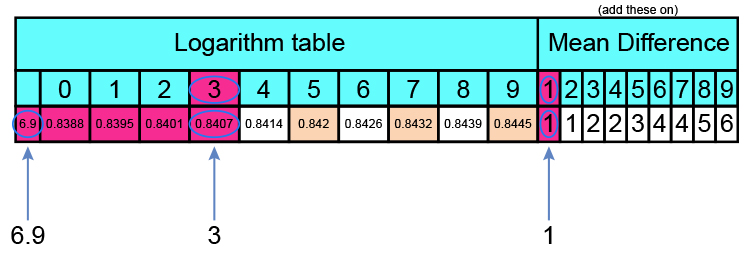
Using the log table convert 69.31 multiply 57.43 to a powered number first look for 69.31

`0.8407 + 1` (to the last digit) = `0.8408`

Log table of `6.931 = 0.8408`

So `69.31 = 10^1\times10^0.8408`

`69.31 = 10^1.8408` (adding indices)

Look up `57.43`

Logs only work between `1` and `10`

And so `57.43` is `10^1\times5.743`

So we look up `5.743`

Here is part of the log table:

Secondly look up 57.43 on the log table

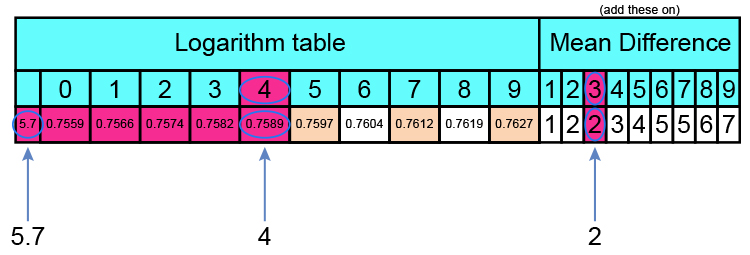
`0.7589 + 2` (to the last digit) = `0.7591`

Log table of `5.743 = 0.7591`

So `57.43 = 10^1\times 10^0.7591`

`57.43 = 10^(1.7591)` (adding indices)