Upper and lower boundary multiplication
If you are looking to multiply two quantities and you need to know the possible limits upper boundary and lower boundary use logic.
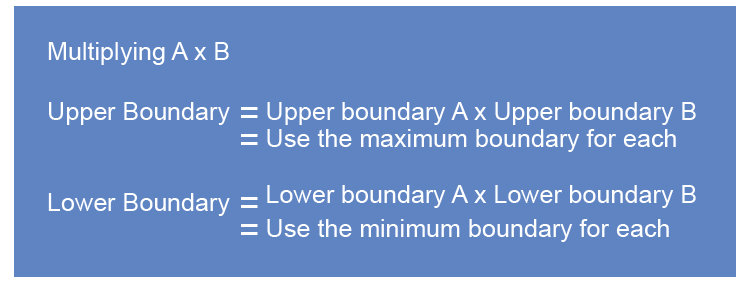
NOTE:
1. Upper boundary `=` Upper boundary `Atimes` Upper boundary `B`
Multiplying the two largest possible boundaries will provide you with the biggest quantity possible.
Upper boundary Big`times`Big`=` Largest amount
2. Lower boundary `=` Lower boundary `Atimes` Lower boundary `B`
Multiplying the two smallest possible lower boundaries will provide you with the smallest quantity possible.
Lower boundary `=` Smallest`times`Smallest`=`Smallest amount
Example 1
The area of a floor that needs painting has been measured as `5mtimes4m` to the nearest metre. What are the minimum and maximum areas that this could be?
This question is a multiplication question but the first thing you need to do is find the maximum and minimum values of each number.
The Upper boundary and lower boundary for `5m` would be:
`5m` to the nearest `1m`
`ul5` underline the last significant number.
`ul5\.0` look next door
`0` `5` or more raises the score. So `4.5` would raise to `5`.
`0` four or less just ignore `5.499` it would ignore and stay at `5`.
This is simplified to `5.5`
So the upper and lower boundary of `5m` is `5.5m` and `4.5m`.
The upper boundary and lower boundary of `4m` would be:
`4m` to the nearest `1m`
`ul4` underline the last significant number.
`ul4\.0` look next door
`0` `5` or more raises the score. So `3.5` would raise to `4`.
`0` four or less just ignore `4.499` it would ignore and stay at `4`.
This is simplified to `4.5`
So the upper and lower boundary of `4m` is `4.5m` and `3.5m`.
Summary
Upper and lower boundary of `5m` is `5.5m` and `4.5m`
Upper and lower boundary of `4m` is `4.5m` and `3.5m`
So the lowest area dimensions would be the minimum boundary of each which is:
| `4.5` | `xx` | `3.5` | `=15.75m^2` |
| Small | Small |
The highest area dimensions would be the maximum boundaries of each which is:
| `5.5` | `xx` | `4.5` | `=24.75m^2` |
| Big | Big |
Answer: The minimum and maximum this area could be is `15.75m^2` and `24.75m^2`.
Example 2
The width of a rectangle was measured as `50cm` and was correct to `2` significant figures and the length was measured as `120cm` correct to 3 significant figures. What are the lower and upper bound areas that this rectangle could be?
This question is a multiplication question but the first thing you need to do is find the maximum and minimum values of each number.
The Upper boundary and lower boundary for `50cm` to `2` significant figures would be:
`50cm` to `2` significant figures
`5ul0` underline the last significant number.
`5ul0\.0` look next door
`0` `5` or more raises the score. So `49.5` would raise to `50`.
`0` four or less just ignore `50.49dot9` it would ignore and stay at `50`.
This is simplified to `50.5`
So the upper and lower boundary of `50cm` is `50.5cm` and `49.5cm`.
The upper boundary and lower boundary of `120cm` to `3` significant figures would be:
`120cm` to `3` significant figures.
`12ul0` underline the `3^(rd)`significant number.
`12ul0\.0` look next door
`0` `5` or more raises the score. So `119.5` would raise to `120`.
`0` four or less just ignore `120.499` it would ignore and stay at `120`.
This is simplified to `120.5`
So the upper and lower boundary of `120cm` is `120.5cm` and `119.5cm`.
Summary
Upper and lower boundary of `50cm` is `50.5cm` and `49.5cm`
Upper and lower boundary of `120cm` is `120.5cm` and `119.5cm`
So the lowest area dimensions would be the minimum boundary of each which is:
| `49.5` | `xx` | `119.5` | `=5915.25cm^2` to 2 D.P. |
| Small | Small |
The highest area dimensions would be the maximum boundaries of each which is:
| `50.5` | `xx` | `120.5` | `=6085.25cm^2` to 2 D.P. |
| Big | Big |
Answer: The minimum and maximum this area could be is `5915.25cm^2` and `6085.25cm^2`.




