Adjacent and the world of cosine

NOTE:

Only look at this if you want to know where cosine comes from.

Mathematicians built a world of right-angled triangles where the hypotenuse was always 1m long.

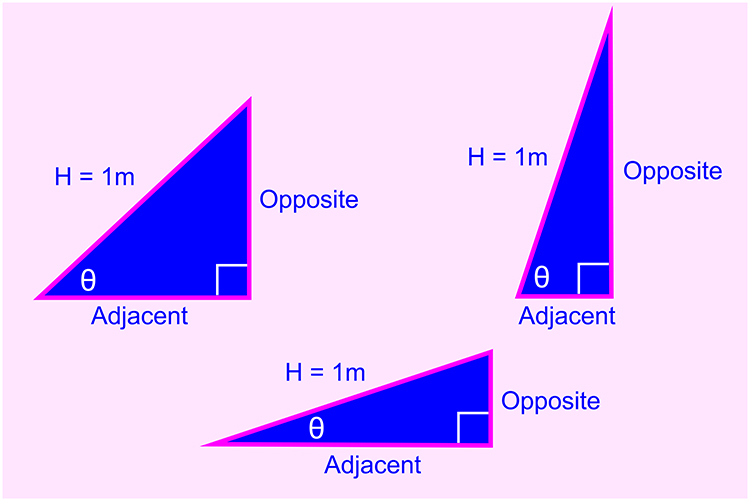
The mathematicians lifted a 1 metre stick up in one degree intervals and measured how far the end lifted off the floor

The mathematicians lifted a 1metre stick up in one degree intervals and measured how far the adjacent length along the floor was.

Fore each degree they measured the opposite side which changed slowly

And for each degree they measured the length of the adjacent side of the triangle and plotted it on a graph. (The red triangle above shows the stick at 45 degrees, and the adjacent side is 0.707 metres along the floor.)

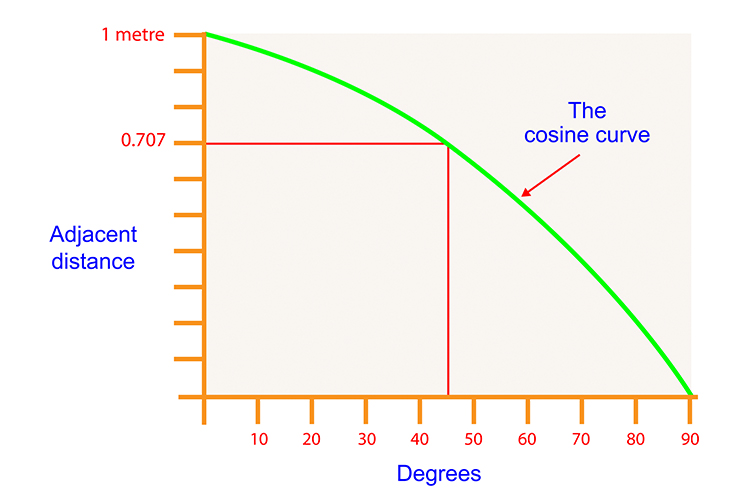
Each distance can be shown on a graph, you will see a curve form, this is the cosine curve

The length of the adjacent side for each degree increase is called the table/chart of cosine (angle) and is as follows:

NOTE:

Mathematicians can work this cosine curve above into a mathematical formula.

Angle		Adjacent distance (or cosine)
`0^@`		`1 \ metre`
`1^@`		`0.99985 \ metres`
`2^@`		`0.99939 \ metres`
`3^@`		`0.99863 \ metres`
`4^@`		`0.99756 \ metres`
`5^@`		`0.99619 \ metres`
`6^@`		`0.99452 \ metres`
`7^@`		`0.99255 \ metres`
`8^@`		`0.99027 \ metres`
`9^@`		`0.98769 \ metres`
`10^@`		`0.98481 \ metres`
`11^@`		`0.98163 \ metres`
`12^@`		`0.97815 \ metres`
`13^@`		`0.97437 \ metres`
`14^@`		`0.97030 \ metres`
`15^@`		`0.96593 \ metres`
`16^@`		`0.96126 \ metres`
`17^@`		`0.95630 \ metres`
`18^@`		`0.95106 \ metres`
`19^@`		`0.94552 \ metres`
`20^@`		`0.93969 \ metres`
`21^@`		`0.93358 \ metres`
`22^@`		`0.92718 \ metres`
`23^@`		`0.92050 \ metres`
`24^@`		`0.91355 \ metres`
`25^@`		`0.90631 \ metres`
`26^@`		`0.89879 \ metres`
`27^@`		`0.89101 \ metres`
`28^@`		`0.88295 \ metres`
`29^@`		`0.87462 \ metres`
`30^@`		`0.86603 \ metres`
`31^@`		`0.85717 \ metres`
`32^@`		`0.84805 \ metres`
`33^@`		`0.83867 \ metres`
`34^@`		`0.82904 \ metres`
`35^@`		`0.81915 \ metres`
`36^@`		`0.80901 \ metres`
`37^@`		`0.79864 \ metres`
`38^@`		`0.78801 \ metres`
`39^@`		`0.77715 \ metres`
`40^@`		`0.76604 \ metres`
`41^@`		`0.75471 \ metres`
`42^@`		`0.74314 \ metres`
`43^@`		`0.73135 \ metres`
`44^@`		`0.71934 \ metres`
`45^@`		`0.70711 \ metres`
`46^@`		`0.69466 \ metres`
`47^@`		`0.68200 \ metres`
`48^@`		`0.66913 \ metres`
`49^@`		`0.65606 \ metres`
`50^@`		`0.64279 \ metres`
`51^@`		`0.62932 \ metres`
`52^@`		`0.61566 \ metres`
`53^@`		`0.60182 \ metres`
`54^@`		`0.58779 \ metres`
`55^@`		`0.57358 \ metres`
`56^@`		`0.55919 \ metres`
`57^@`		`0.54464 \ metres`
`58^@`		`0.52992 \ metres`
`59^@`		`0.51504 \ metres`
`60^@`		`0.5 \ metres`
`61^@`		`0.48481 \ metres`
`62^@`		`0.46947 \ metres`
`63^@`		`0.45399 \ metres`
`64^@`		`0.43837 \ metres`
`65^@`		`0.42262 \ metres`
`66^@`		`0.40674 \ metres`
`67^@`		`0.39073 \ metres`
`68^@`		`0.37461 \ metres`
`69^@`		`0.35837 \ metres`
`70^@`		`0.34202 \ metres`
`71^@`		`0.32557 \ metres`
`72^@`		`0.30902 \ metres`
`73^@`		`0.29237 \ metres`
`74^@`		`0.27564 \ metres`
`75^@`		`0.25882 \ metres`
`76^@`		`0.24192 \ metres`
`77^@`		`0.22495 \ metres`
`78^@`		`0.20791 \ metres`
`79^@`		`0.19081 \ metres`
`80^@`		`0.17365 \ metres`
`81^@`		`0.15643 \ metres`
`82^@`		`0.13917 \ metres`
`83^@`		`0.12187 \ metres`
`84^@`		`0.10453 \ metres`
`85^@`		`0.08716 \ metres`
`86^@`		`0.06976 \ metres`
`87^@`		`0.05234 \ metres`
`88^@`		`0.03490 \ metres`
`89^@`		`0.01745 \ metres`
`90^@`		`0 \ metres`