Sine examples
Example 1
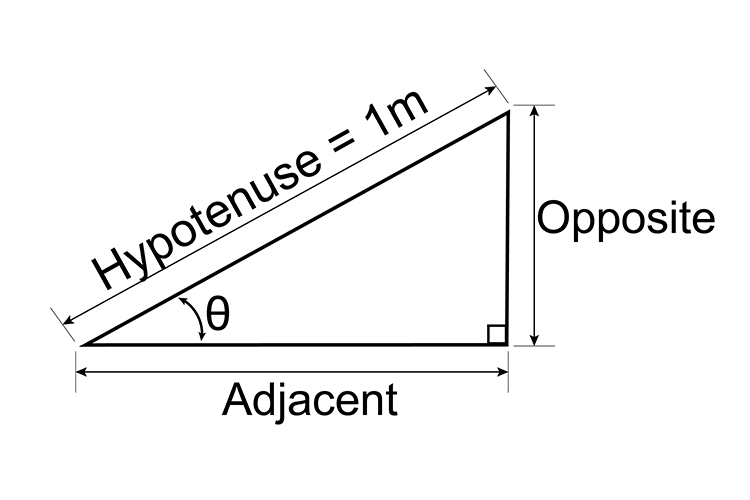
If the hypotenuse is 1 metre long and θ=35∘, how long is the opposite side?
Look up on the table/chart of sine (angle) for 35∘or look up sine 35∘ on a calculator.
|
Angle |
Opposite distance (or sine) |
|
|
35∘ |
0.5736metres |
Answer:
Hypotenuse = 1 metre,
θ=35∘,
Therefore, Opposite = 0.5736 metres.
Example 2
If the hypotenuse is 1 metre long and θ=45∘, how long is the opposite side?
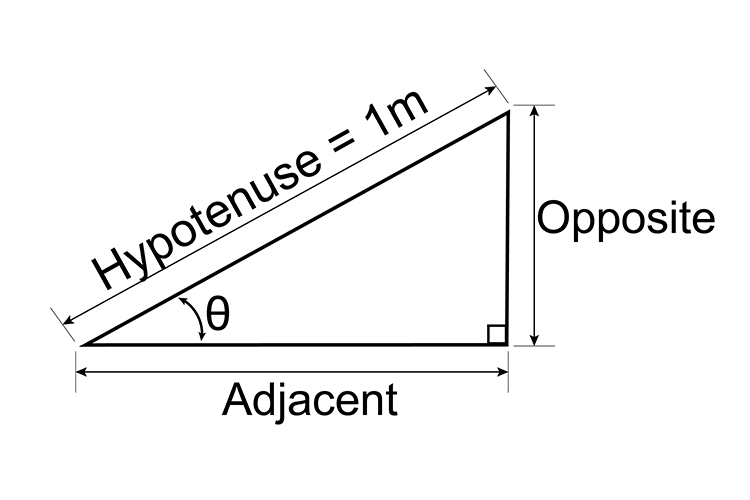
Look up on the table/chart of sine (angle) for 45∘ or look up sine 45∘ on a calculator.
|
Angle |
Opposite distance (or sine) |
|
|
45∘ |
0.7071metres |
Answer:
Hypotenuse = 1 metre,
θ=45∘,
Therefore, Opposite = 0.7071 metres.
Example 3
If the hypotenuse is 1 metre long, and the opposite side is 0.8 metres long, what is the angle θ ?

Look up on the table/chart of sine (angle) for 0.8 metres and find the angle.
|
Angle |
Opposite distance (or sine) |
|
|
? |
0.8metres |
The nearest = 54° = 0.809 metres.
But on a calculator if you put in 0.8 and press inverse sin (sin-1), this gives you 53.13° (which is more accurate).
Example 4
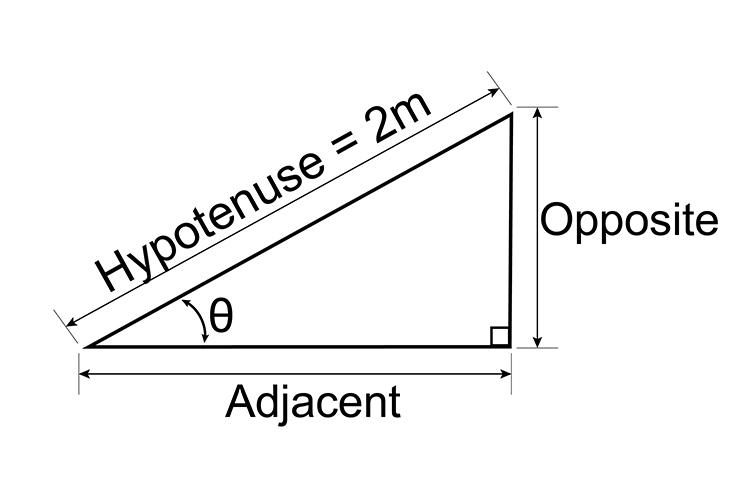
If the hypotenuse is 2 metres long and θ=45∘, how long is the opposite side?
Look up on the table/chart of sine (angle) for 45∘ or look up sine 45∘ on a calculator.
|
Angle |
Opposite distance (or sine) |
|
|
45∘ |
0.7071metres |
But 0.7071 is for a 1m hypotenuse.
For a hypotenuse of 2m, the opposite would be twice as long.
Therefore 0.7071×2=1.4142
Answer:
Hypotenuse = 2 metres,
θ=45∘,
Therefore opposite = 1.4142 metres.




