Sketch the bell curve

When you are told the standard deviation, the mean and the highest frequency of a set of data you should be able to sketch a pretty accurate reflection of events.

Here are examples:

Example 1

Farmers were asked how many people they employe.

The mean was 6

60 of the farmers had 6

and the standard deviation = 2

To build the graph draw the `x` and `y` axis and locate the top of the graph.

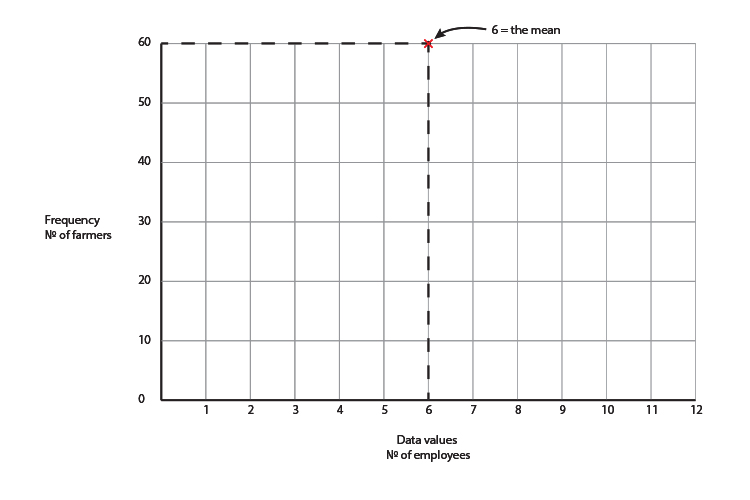First draw a straight mean line If 6 is the mean that is the top of the graph and because 60 of the farmers had 6 employees, 60 must be the highest frequency.

Now we have located the top of the graph. We can now work out the impact of a standard deviation which is 2.

2 either side of the mean = 68%

4 either side of the mean = 95%

6 either side of the mean = 99.7%

Plot the standard deviation positions on the graph

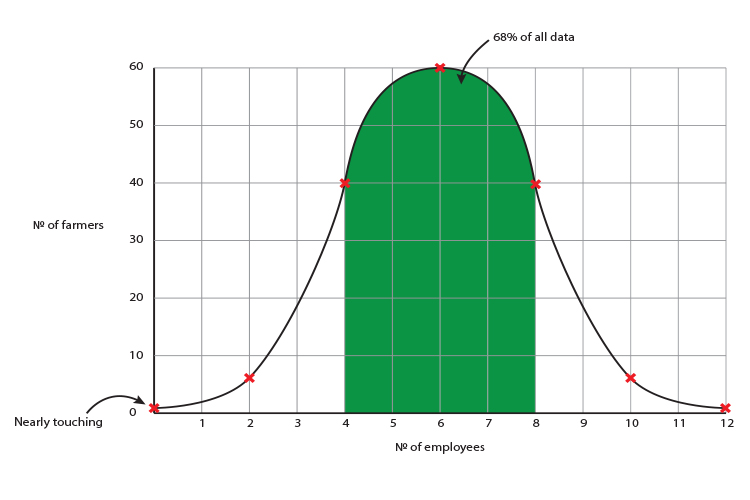
68 percent of data should always be shown in the 1SD position

This graph gives you a good rough guide as to how the data should be interpreted.

Example 2

Students were asked how many hours of TV they watched per week.

80 students watched 30 hours per week and 30 hours was also the mean. The standard deviation was 5 hours.

Draw a rough sketch of the bell curve:

To build the graph draw the `x` and `y` axis and locate the top of the graph.

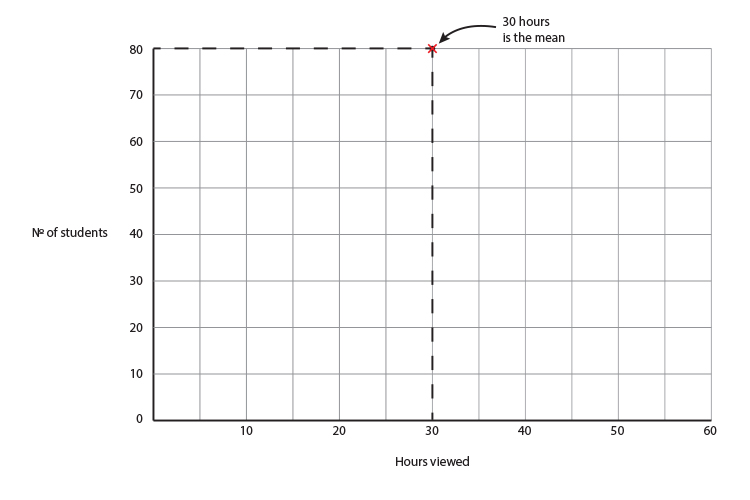Draw the mean for this example If 30 hours is the mean that is the top of the graph and because 80 students watched 30 hours, 80 must be the highest frequency.

Now we have located the top of the graph we can work out the impact of a standard deviation which is 5 hours.

5 hours either side of the mean = 68%

10 hours either side of the mean = 95%

15 hours either side of the mean = 99.7%

Plot the standard deviation positions on the graph

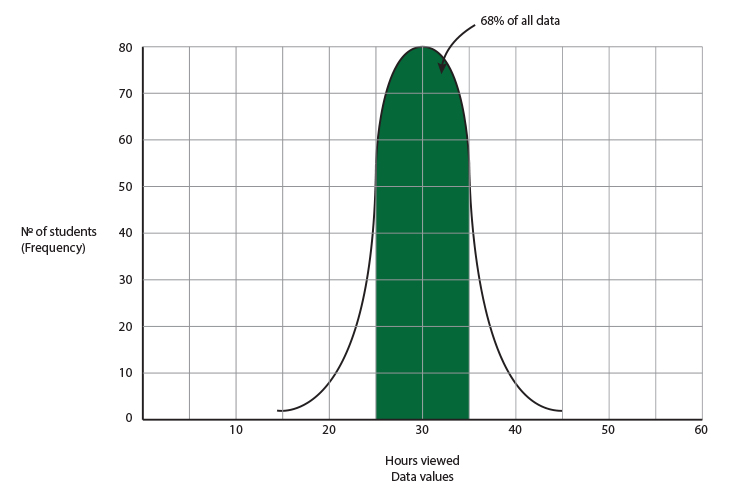Even though the bell curve id more abrupt than example 1, 68 percent of data should be displayed in the middle This graph gives you a rough guide as to how the data should be interpreted.