Working out the critical angle

The critical angle (where `theta\ \o\u\t=90^circ`) can be worked out for different media using the following process.

Use

`sin\ \i\n\theta=1/(n(i\n))`

because

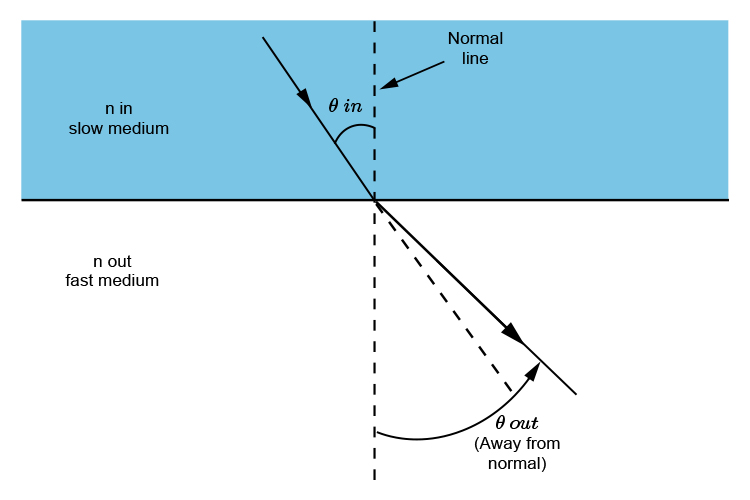
Remember FAST SOFA (slow in fast out away from the normal line)

and remember

`(sin\ \i\n\theta)/(sin\ \o\u\t\theta)=(n\ \(o\u\t))/(n\ \(i\n))`

but because `theta\ \o\u\t` is at `90^circ` `(sin90=1)` and `n\ \o\u\t` is 1 (for air) . . .

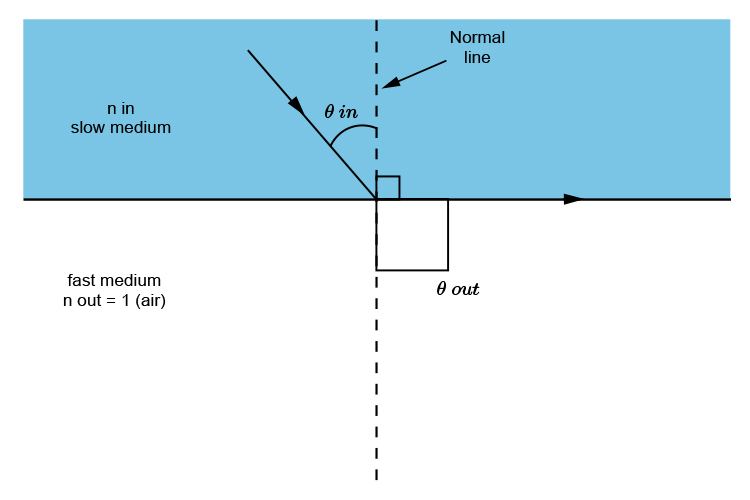
. . . the formula becomes

`(sin\ \i\n\theta)/1=1/(n\ \(i\n))`

and simplified

`sin\ \i\n\theta=1/(n\ \(i\n))`

Water to air

(water `n=1.3`)

`sin\ \i\n\theta=1/1.3=0.769`

`theta=sin^-1\0.769`

`theta=50.26~~50^circ`

Glass to air

(Glass `n=1.5`)

`sin\ \i\n\theta=1/1.5=0.66dot 6`

`theta=sin^-1\0.66dot 6`

`theta=41.81~~42^circ`

Diamond to air

(Diamond `n=2.417`)

`sin\ \i\n\theta=1/2.417=0.4137`

`theta=sin^-1\0.4137`

`theta=24.44~~24^circ`

Conclusion

The critical angle for