General exam questions

Example 1

A baby’s weight increases by `7%` over a month from birth to `3.9kg`. What was the baby’s weight at birth?

NOTE:

A `7%` increase on `100%` is `107%`

Multiply both sides by `3.9` to get `x` on its own (remember - what you do to one side you do to the other).

`(100times3.9)/107=(xtimescancel3.9)/cancel3.9`

`(100times3.9)/107=x`

    `x=(100times3.9)/107`

    `x=3.64`

Answer: The baby’s weight at birth was `3.64kg`

Example 2

John buys a drill for `£150.00` inc sales tax and James buys the same drill using a trade account for `£120.00` exc sales tax. If sales tax is charged at `20%` how much tax did John and James pay and who paid the least in total for the drill?

First work out what James paid.

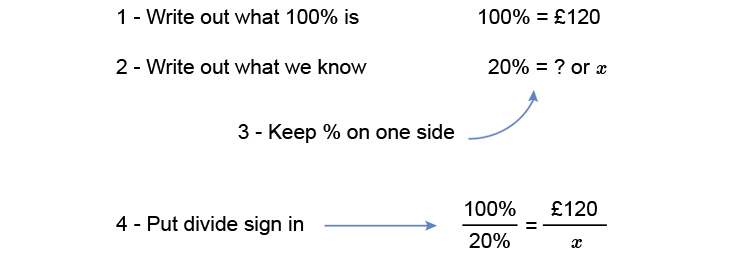
Multiply both sides by `x` to get `x` on its own

`(100timesx)/20=(120timescancelx)/cancelx`

`(100x)/20=120` 

 
Multiply both sides by `20` to get `x` on its own

`(100xtimescancel20)/cancel20=120times20`

`100x=120times20`

 
Divide both sides by `100` to get `x` on its own

   `(cancel100x)/cancel100=(120times20)/100`

`x=(120times20)/100`

`x=(24cancel00)/(1cancel00)`

`x=24`

James paid `£24.00` tax on his drill and a total of `£120.00 + £24.00 = £144.00`

Now lets work out what John paid.

NOTE:

`£150.00` is including the `20%` sales tax so is equal to `120%`?

Multiply both sides by `150.00` to get `x` on its own

  `(100times150)/120=(xtimescancel150)/cancel150`

  `(100times150)/120=x`

`x=(100times150)/120`

`x=125` 

John paid `£125.00` excluding sales tax for his drill, so the sales tax he paid was:

`£150.00 - £125.00 = £25.00`

Answer: John paid `£25.00` sales tax on his drill and `£150.00` in total

              James paid `£24.00` in sales tax on his drill and `£144.00` in total

              So James paid less for his drill.