Inequalities and graphs example 2
Graph the following inequality:
4y-x≤4
Add x to both sides to get y on its own.
4y-x≤4
4y-x+x≤4+x
4y≤4+x
Divide both sides by 4 to get y on its own.
4y4≤44+x4
y≤1+x4
y≤x4+1
First, let's calculate some points on the graph.
| x=-2 | then | y≤(-24)+1=(-12)+1=12 | ||
| x=-1 | then | y≤(-14)+1=34 | ||
| x=0 | then | y≤(04)+1=1 | ||
| x=1 | then | y≤14+1=114 | ||
| x=2 | then | y≤24+1=112 |
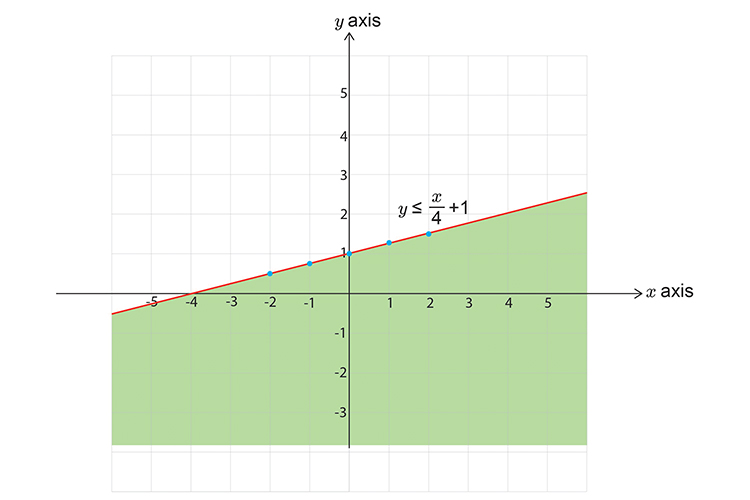
Now draw a graph and mark on the above points. Don’t forget to use a SOLID line.
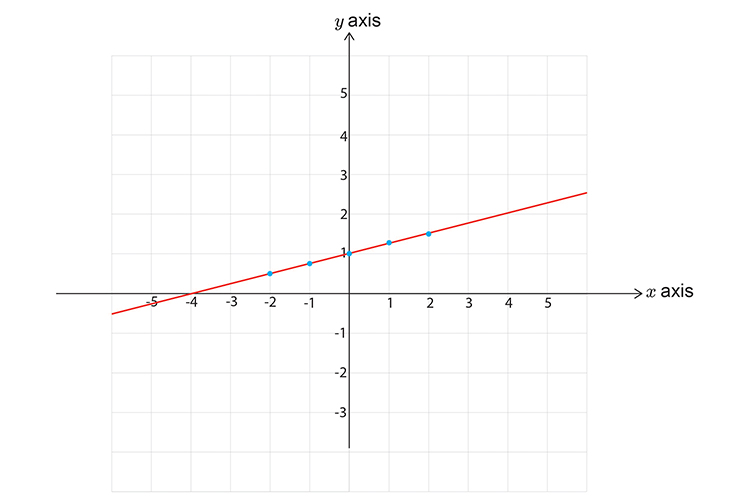
Now pick a point not on the line.
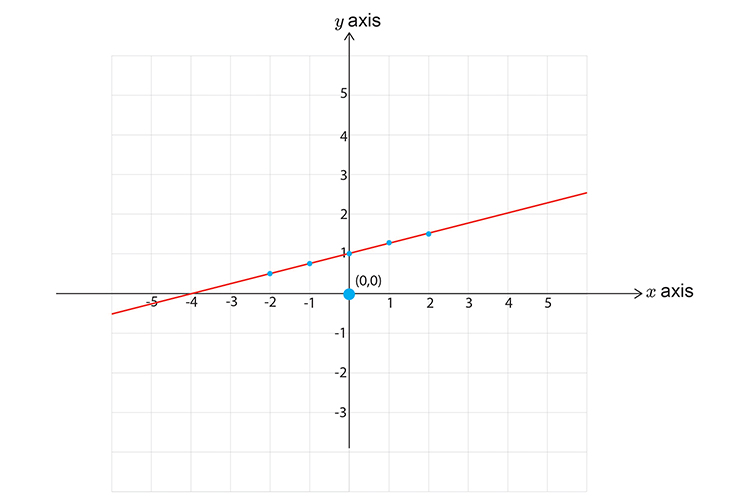
Choose x=0 and y=0
y≤x4+1
0≤04+1
0≤1
This read 0 is less than or equal to 1. This is obviously correct.
This DOES satisfy the inequality.
SHADE IT.